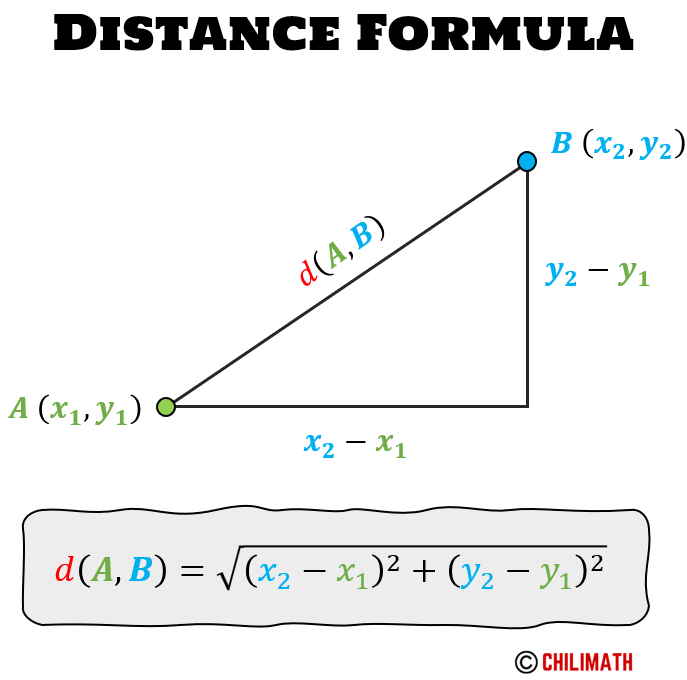
Here are ten (10) practice exercises about the distance formula. As you engage with these problems, my hope is that you gain a deeper understanding of how to apply the distance formula. Good luck!
Problem 1: How far is the point [latex]\left( < – 4,6>\right)[/latex] from the origin?
[latex]\color2\sqrt [/latex] units
Problem 2: Find the distance between the points [latex]\left( \right)[/latex] and [latex]\left( \right)[/latex]. Round your answer to the nearest hundredth.
Problem 3: Find the distance between the points on the XY-plane. Round your answer to one decimal place.
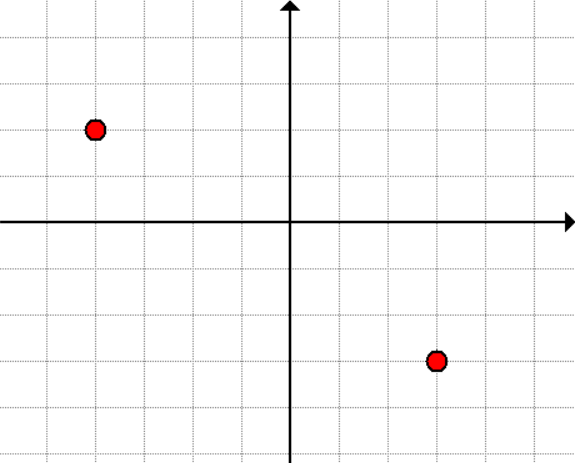
Problem 4: Determine the distance between points on the coordinate plane. Round your answer to two decimal places.
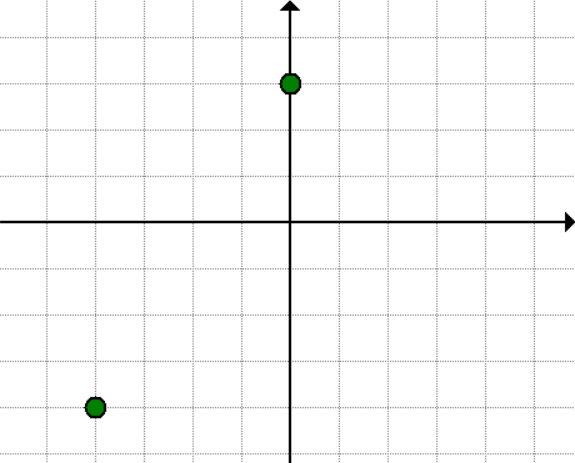the coordinate plane. the points are (0,3) and (-4,-4)." width="575" height="463" />
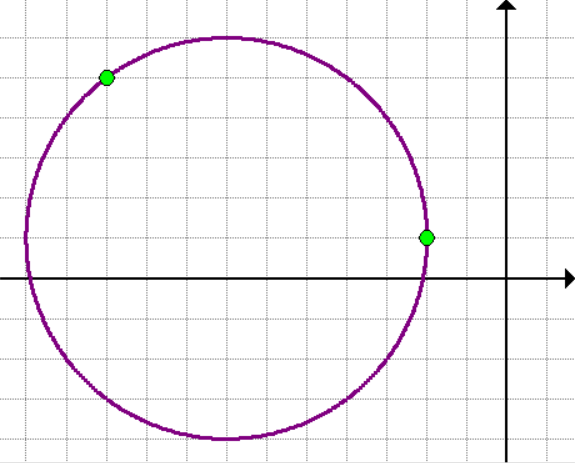
Problem 5: The chord of a circle has endpoints as shown below (in green dots). What is the length of the chord?
[latex]\color4\sqrt 5[/latex] units
Problem 6: The diameter of a circle has endpoints as shown below (in red dots). What is the length of the diameter?
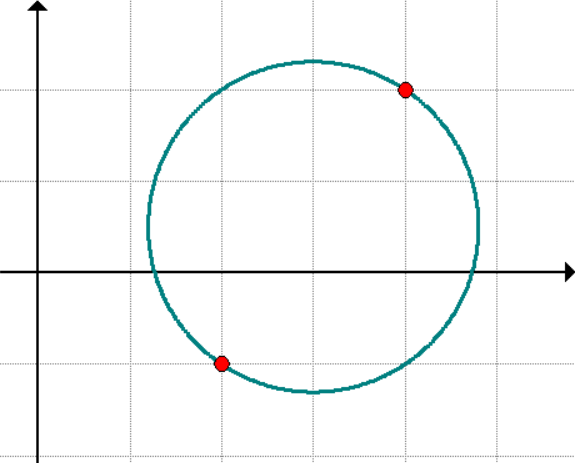
[latex]\color\sqrt 13[/latex] units
Problem 7: Find the two points on the x-axis that are [latex]15[/latex] units away from the point [latex]\left( \right)[/latex].
[latex]\left( \right)[/latex] and [latex]\left( < – 14,0>\right)[/latex]
Problem 8: Find the two points found on the y-axis which are [latex]25[/latex] units from the point [latex]\left( \right)[/latex].
[latex]\left( \right)[/latex] and [latex]\left( \right)[/latex]
Problem 9: Find the values of [latex]\colork[/latex] such that the points [latex]\left( <<\colork>, – 1> \right)[/latex] and [latex]\left( \right)[/latex] have a distance of [latex][/latex] units.
[latex] = – 7[/latex] and [latex] = 17[/latex]
Problem 10: Find the values of [latex]\colorm[/latex] such that the points [latex]\left( <<\colorm>,3> \right)[/latex] and [latex]\left( <1,<\colorm>> \right)[/latex] are [latex]10[/latex] units apart.
[latex] = – 5[/latex] and [latex] = 9[/latex]
You might also like these tutorials:
Categories
